Definition :-
Understanding the rates of cooling and solidification in laminar flows down sloping channels is central to predicting the advance of lava flows.
The mechanisms involved include thermal convection and a competition between shear strain rate and the rate of formation of solid at the chilled surface of the flow. We report experiments in which polyethylene glycol wax flows in a laminar fashion down an inclined, open channel of rectangular cross-section under cold water.
Two distinctly different flow regimes are recognized: ‘tube’ flow in which solidification of the flow surface creates a stationary roof while melt continues to flow through a relatively well-insulated ‘tube’ beneath, and a ‘mobile crust’ regime in which a solid surface crust develops only in the centre of the channel.
In the latter regime the crust is carried down the channel, separated from the walls by crust-free shear regions in which cooling produces only dispersed fragments of solid owing to the effects of shearing. This flow structure is quasi-invariant over a large distance downstream.
We show that thermal convection takes place in organized rolls that have axes aligned with the shear flow, and conclude that transition between the two flow regimes occurs at a critical value of the combined parameter
, where is the ratio of a surface solidification timescale to a shearing timescale , and are the flow depth and centreline surface velocity in the absence of solidification, {\it Ra} is a Rayleigh number and is a constant.
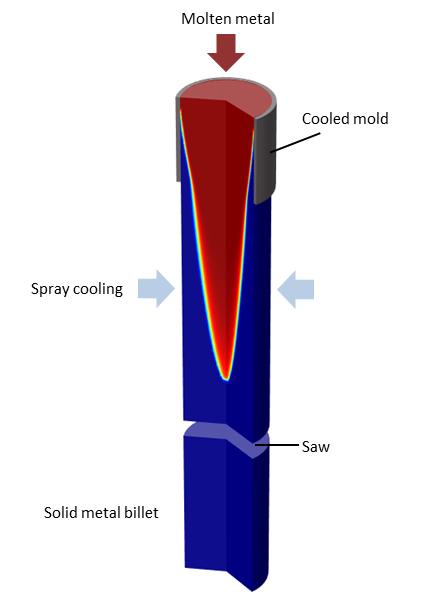
Example: Continuous Casting
Understanding the rates of cooling and solidification in laminar flows down sloping channels is central to predicting the advance of lava flows.
The mechanisms involved include thermal convection and a competition between shear strain rate and the rate of formation of solid at the chilled surface of the flow. We report experiments in which polyethylene glycol wax flows in a laminar fashion down an inclined, open channel of rectangular cross-section under cold water. Two distinctly different flow regimes are recognized: ‘tube’ flow in which solidification of the flow surface creates a stationary roof while melt continues to flow through a relatively well-insulated ‘tube’ beneath, and a ‘mobile crust’ regime in which a solid surface crust develops only in the centre of the channel.
In the latter regime the crust is carried down the channel, separated from the walls by crust-free shear regions in which cooling produces only dispersed fragments of solid owing to the effects of shearing. This flow structure is quasi-invariant over a large distance downstream. We show that thermal convection takes place in organized rolls that have axes aligned with the shear flow, and conclude that transition between the two flow regimes occurs at a critical value of the combined parameter, where is the ratio of a surface solidification timescale to a shearing timescale , and are the flow depth and centreline surface velocity in the absence of solidification, {\it Ra} is a Rayleigh number and is a constant.
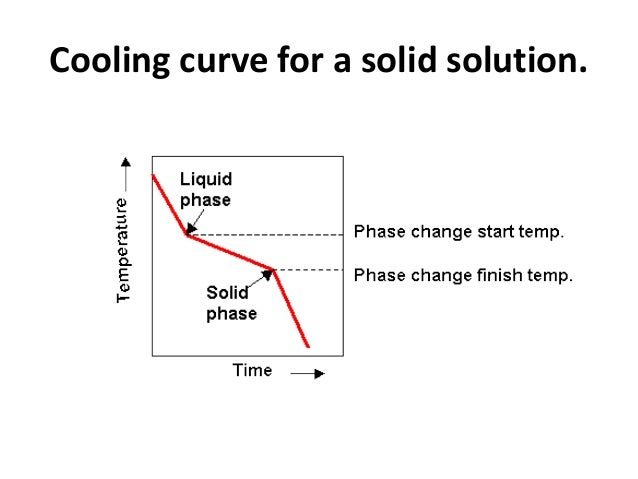
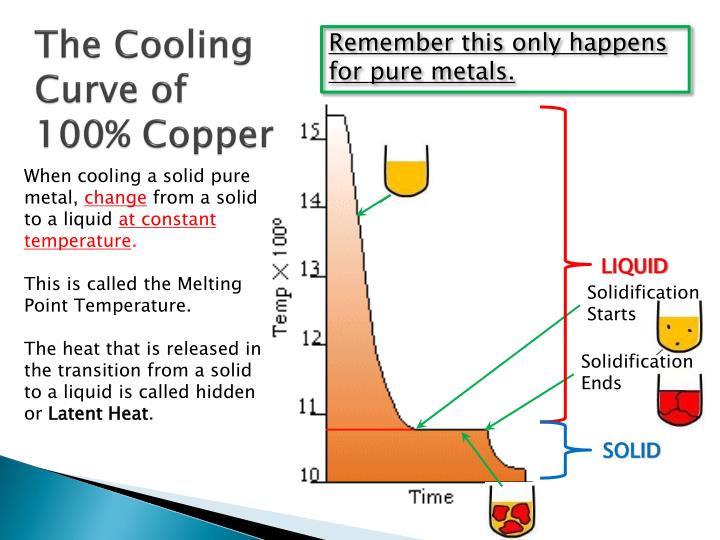
Cooling Curves
Plotting a graph of Temperature and Time as liquid metal cools will produce a. Such graphs serve to illustrate the behaviour of metal as it cools and what changes occur at different temperatures
Typical COOLING CURVE for pure metals will be a stepped graph as shown. Heat is being lost throughout the process but at the point of freezing the temperature doesn't fall because the freezing process will liberate heat at exactly the same rate that it is being lost to the surroundings.
This is the result of energy being released when new atomic bonds are formed formed (e.g changing states from Liquid to Solid Lead or Tin).
Phase Change: Cooling and Solidification of Metal :-
Modeling phase change is important for many thermal processes, ranging from the food industry to the metal processing industry. The Heat Transfer Module offers a dedicated interface for modeling the characteristics of phase change. It uses the apparent heat capacity method, which we introduce here.
Example: Continuous Casting
Phase change is a transformation of material from one state of matter to another due to a change in temperature. Phase change leads to a sudden variation in the material properties and involves the release or absorption of latent heat. We can use the Heat Transfer Module to model this type of phase change. Let’s start with an example.
No comments:
Post a Comment